Goal
Understand fundamentals about the spectrum, harmonics and overtones.
The Spectrum
We saw in the prior lesson that all sound can be broken down into a set of sine and cosine waves via the FFT. These collections of waves are referred to as the spectrum.

A spectrogram is a type of graph that shows us frequency vs magnitude. Some are 3d, others are 2d. Below is one that is 3d.
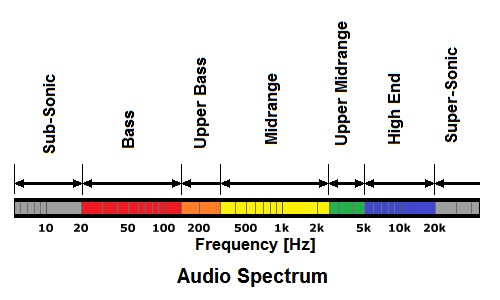
A group of frequencies consider together is called a frequency band. For example 100Hz to 300Hz could be a frequency band. Many multi-band plugins split the signal from the wide band, the band with all frequencies, into the lows, mids, and highs. Many people have different ways of splitting up the signal to suite their purpose.
Harmonics
Before we can dive into frequency bands we must learn some new principles when dealing with frequency, namely that when playing a sound you don't hear a single sine wave, you hear a spectrum. A spectrum is a collection of sine waves, it could be for 1 sound or multiple sounds combined into 1 file. A spectrum specific to a sound is called a timbre (pronounced TAM-ber).
Questions to consider
Consider a flute playing the note C. Now a tuba also plays the note C.
What is the difference between them?
How can we tell them apart despite them playing the same note?
How do we even determine they are playing the same note?
The Fundamental
In a timbre there are some commonalities in nearly all sounds that can play a pitch:
The lowest frequency in the spectrum is the loudest.
Other frequencies in the spectrum are related to or can be derived from the lowest frequency in special ways.
Because of the role the lowest frequency plays in tonal sounds it is called the fundamental. If a sound has an obvious fundamental then it typically is able to play pitched music. Flutes, trombones, and piano all feature strong fundamentals. Cymbals, most drums, and noises like car door closing or pots and pans clashing do not feature strong fundamentals and so struggle to play things like melodies. They are non-tonal.
Harmonics
From the fundamental we can derive the harmonics. If you take the frequency of the fundamental and multiply it by 1 you get the first harmonic. In this case it is just the fundamental but this is why the fundamental is also called the first harmonic. Now lets assume the fundamental is 100Hz. If we multiply this fundamental by 2 we get the second harmonic: 200Hz. If we multiply by 3 we get the third fundamental 300Hz, and so on ... Multiplying the fundamental by the harmonic number you can get any harmonic.
These harmonics carry with them ratios to the fundamental. The simpler this ratio the more easily we process it and "make sense" of it.
Example:
Consider 100Hz and its second harmonic 200Hz. 100Hz has twice the wavelength of 200Hz or in other words its ratio is 2:1. This ratio describes how long it takes for the waves to "line up" again. For the fundamental to line up again it must cycle only once, but the 200Hz or second harmonic, must cycle twice to line up with the first. This is where interval ratios come from. You can see it as This is the next simplest ratio is the fundamental against itself which has a ratio of 1:1 taking first in level of simplicity. You can see it as:
Each harmonic has a ratio to the fundamental. The third harmonic is 3:1, the fourth is 4:1 and so on.

Each harmonic is individually a sine wave. When we put the harmonics together they become more complex sounds. Complex sounds have different sets of harmonics with each harmonic having different amplitudes and phase.
Overtones
Overtones are literally just tones that occur above or "over" the fundamental. A harmonic is an overtone, but not all overtones are harmonics. If a sound contains a frequency that is not some multiple of the fundamental, meaning it is not a harmonic, then it is called an overtone. Overtones may sound "inharmonic" and result in more complex textures because their ratios to the fundamental can be very complex. For example 2.3352455:1 is not exactly straight forward to our brains. There is a great video on this topic that tries to determine how musical a sound is using number theory:
Because of how the fundamental is defined the first harmonic is the fundamental because it is one of itself, but it is not the first overtone because the fundamental cannot be higher than itself. The legend Leonard Bernstein has a video on this:
The amazing part about our brains is that we principally interpret what note is being played by what the fundamental is and we use the harmonics and overtones to determine the timbre of the sound. If I played for you two sine waves and made them distinct enough melodically you would hear two "instruments" or two individually melodic lines. However if I made one an octave apart from the other you would hear 1 instrument playing one line instead of two! I could add another sine wave as the third harmonic and so on and your brain would group it automatically as part of 1 sound. Instead of hearing a bunch of independent sine waves you hear 1 "sound".
This ability to separate sound is fundamental to our ability to interpret music, speech and a many other aspects of sound. Could you imagine hearing 2 voice and not being able to separate them because all you hear is a jumbled mess of sine waves? No, our brain is incredible and knows exactly how to hear both voices and tell each voice apart, not only this it can tell the meaning of what is being said. Your ability to dissect sounds as you hear them will develop through careful ear training but as you get better you will be able to hear "pieces" of sounds you did not recognize before. This is because before you always just let your brain sort it out without focusing on the process of what exactly it was doing.
Questions
Determine the 5th harmonic of 300Hz.
Determine the 2nd harmonic of 1Hz.
Determine the 10th overtone of 100Hz. Explain.
Music theory uses interval ratios to describe the distance between two notes. A perfect fifth has a ratio of 3:2. If the fundamental is 100Hz what is the frequency of the fifth?
Can a harmonic have a wavelength bigger than the fundamental? Why?
A wave has a fundamental of 110Hz, what is the wavelength of its 3 harmonic?
A waves 4th harmonic is found to be 800Hz, what is its fundamental?
A wave has a wavelength of 11.3ft, what is the the fundamental if it is the 3 harmonic?
For the harmonic series: 21Hz 42Hz 63Hz 84Hz 105Hz 123Hz, is the series harmonic?
The Harmonic Series
We can get any harmonic from using the formula:
If we let n start at 1 and go up to infinity we obtain the harmonic series. Note this harmonic series can be described by its interval ratios. Namely: 1:1, 2:1, 3:1, ... n:1. This leads us to the math series referred to as the harmonic series:
The giant thing that looks like an E is the greek letter sigma and means to repeatedly perform an addition so it is called a "summation". On the bottom it says where the variable that changes after each sum is on the bottom and on top it says when to stop. If we expand it out we get an infinite sum! In the real world this particular series appears often as we have just stumbled across it by using frequency!
This series can churn out a lot of the basic waveforms.
Wave | Spectrum | Harmonic Series |
|---|---|---|
Sine |  | A single frequency. The amplitude is 1 and the phase is 0°. |
Saw |  | Contains every harmonic. Each harmonic has the amplitude of its reciprocal, so the fundamental is 1, the second harmonic is 1/2 as loud the third is 1/3 as loud ect... There are no phase shifts. |
Square |  | Contains every odd harmonic. Each harmonic has the amplitude of its reciprocal, so the fundamental is 1, the third harmonic is 1/3 as loud the fifth is 1/5 as loud ect... There are no phase shifts. |
Triangle |  | Contains every odd harmonic. Each harmonic has the amplitude of its reciprocal squared, so the fundamental is 1, the second harmonic is 1/4 as loud the third is 1/9 as loud ect... Every other harmonic has a phase shift of 180°. |
White Noise |  | Equal energy per frequency. Each frequency is equally likely to occur. |
Pink Noise |  | Equal energy per octave. The power spectrum of pink noise drops by 3dB per octave. We hear more similarly to pink noise. |
Often we are not just concerned with the fundamental but also its harmonics.
Intervals
Using the spectrum we can talk about the intervals between notes. The observation of these intervals is what leads to the various tuning systems. For the time we are only concerned with the intervals.
What is an interval?
An interval is a ratio of distance between two notes. It is not just the distance itself because we hear pitch in a "timesy" kind of way. For example, if I played for you 100Hz and then 200Hz they would sound like the same note just higher. If I then played 400Hz and 800Hz the same thing would occur. It is not the distance that matters, because 100 to 200 has a distance of 100, but 400 to 800 has a distance of 400! It is the ratio that matters.
For intervals we are asking the question: "How many on one cycle does it take to line up with another?". For instance it takes 1 cycle of the fundamental and 2 of its octave for them to line up. For a perfect 5th it takes 3 cycles of the fifth and 2 of the fundamental to line up.
The Octave
Perhaps the ratio you will hear mentioned the most is the octave. It is the ratio of 2:1. We care a lot about this ratio because in western harmony all 12 notes occur every octave, so the octave is sort of like a "block" of frequency split into 12 parts, one for each note. Each note we give 100 cents. Because we hear in this "time 2" kind of way the frequencies the 100 cents covers changes depending on the octave. The beauty of 100 cents per note is that to get to the next note you must move 100 cents. Thats it, its linear.
There is a lot we could go into here but we only need to know that the octave is 2:1 and it has a fundamental role in music theory as well as acoustics. It's tied to the very way we perceive pitch. Pitch is different than frequency in that pitch is how humans hear frequency. 100Hz and 200Hz are certainly different frequency, and to be sure they are different pitches as well, but they belong to the same "class" of pitch. The note "A 440" and "A 880" are both part of the pitch class A. Why? Because that is how humans hear it.
If I wanted all "A's" in existence I could just multiply or divide by 2 to get them. 220Hz, 110Hz, 55Hz ect.. are all "A's". Similarly 440Hz, 880Hz, 1760Hz, ect ... are also all "A's". Note that each octave contains twice as many frequencies as the one before it because of this "times 2" way of hearing! Yet they contain the same 12 notes in western harmony! It is here I can welcome you to the world of tuning but I will only go this far. If your interested in going further you will have to research it on your own.
Questions
What is frequency is 3 octaves above 220Hz?
What is frequency is 3 octaves below 220Hz?
What is the second harmonic of the frequency 1 octave below 550Hz?
How many frequencies are in the band from 300Hz to the next octave?
Other Intervals
There are many other intervals. The main ones used in western harmony can be used to get the 12 notes and they are:
| Half Step Delta | Interval Name | Interval Ratio |
|---|---|---|
| 0 | Perfect 1 | 1:1 |
| 1 | Minor 2 | 16:15 |
| 2 | Major 2 | 9:8 |
| 3 | Minor 3 | 6:5 |
| 4 | Major 3 | 5:4 |
| 5 | Perfect 4 | 4:3 |
| 6 | Tritone | 45:32 |
| 7 | Perfect 5 | 3:2 |
| 8 | Minor 6 | 8:5 |
| 9 | Major 6 | 5:3 |
| 10 | Minor 7 | 9:5 |
| 11 | Major 7 | 15:8 |
| 12 | Perfect 8 | 2:1 |
Each of these ratios can be though of as a fraction. When going up we will multiply and when going down we will divide.
Example
Say we want the perfect 5th up from 100Hz. Well the ratio for the perfect fifth is 3:2 and 3/2 is 1.5 so we just multiply 100Hz times 1.5 and we get 150Hz which is the perfect fifth. This is saying for every 3 cycles in 150Hz it will line up with the second cycle in 100Hz. Here it is plotted in Desmos with the red being 100Hz and blue being 150Hz. If you zoom in you will find this to be the case:
Questions
For the fundamental of 100Hz what would be the frequencies of the 12 notes that occur in the octave?
Noise
Noise is one of the things that was very hard to characterize because of its waveform. It looks erratic. However the frequency domain representation has some properties that make it easier to characterize. There are many types of noise and we will only touch on a few.
White Noise
White noise has equal energy per frequency, or in other words each frequency is equally likely to occur. White noise is useful for different measurements and will be mentioned when needed.
Pink Noise
Pink noise has equal energy per octave. Each octave grows having double the frequencies of the previous octave. But it must contain the same amount of energy as the previous octave. This results in each octave getting softer by 3dB. We hear more similarly to pink noise and as such we will also use pink noise in many acoustic measurements.
Wave Approximations
Complex waves can often be simplified to more fundamental wave types. Many start out very complex but end in some periodic wave. For example:
Most drums end in a sinusoidal pattern.
Many brass and string instruments are similar to saw waves.
Many clarinets and other reed instruments are often similar to square waves.
Plucked instruments like piano or guitar often end in a sine wave.
To support this series please consider donating via
paypalor joining the
patreon.