Goal
Understand and construct minor scales.
Minor Scales
Just like major scales, minor scales are a collection of intervals. Minor scales have 3 varieties we must become familiar with. (If you have skipped the major scale lesson please see that lesson as this one builds off of that one.)
- Natural Minor
- Harmonic Minor
- Melodic Minor
Before we dive into those construction methods it is often easier to make the major scale and change it to be “minor”.
Major to Minor
Each minor scale can be made from a major scale with just a few adjustments. Namely in each case we simply lower some of the scale degrees to make them minor.
Natural Minor: Lower 3,6,7
Harmonic Minor: Lower 3,6
Melodic Minor: Up: Lower 3, Down: Lower 3,6,7
Note that each minor scale has a major second. These scales have mostly minor intervals and that is why all these scales are considered minor. (This concept leads to the topic of modes, which you can see as a subset of “scales”).
To make any of these scales then all you must do is make the major version and then lower the appropriate scale degrees.
Example:
Consider D natural minor. To construct such a scale make D major:

The natural minor has a lowered 3, 6, and 7 so we simply lower those and we get:

Boom! D natural minor.
Let's say you wanted a harmonic minor. Then just lower the 3rd and the 6th!

For melodic minor lower the 3rd going up and the 3, 6 and 7 going down.
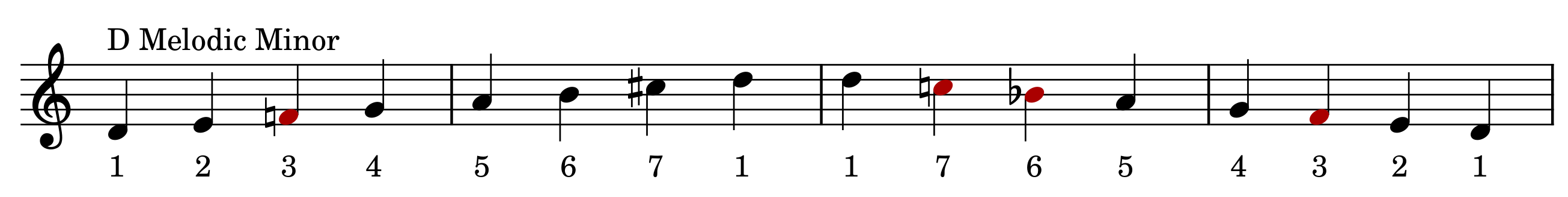
This scale is different going up than going down so be careful!
That is all you must do to make the minor scales.
Properties of the Minor Scales
Each minor scale has certain harmonic properties. In particular a very desirable quality is to keep the leading tone. That half step resolution is very useful. That is why the harmonic minor has a raised 7th. To keep that relationship. After we cover diatonic chord progressions (a later lesson) you will see some of the reasons a raised 7th is useful.
Other Methods of Construction
You can of course construct them from a set of whole and half steps or a set of intervals. The only one you can construct from the circle of 5ths and 4ths (construction method 3 in the major scale lesson) is the natural minor.
Construction Method 1 (Works for all three)
The patterns for minor scales are as follows:
Natural Minor: WHWWHWW (if you look closely this is a shifted version of the major scale!)
Harmonic Minor: WHWWH (W+H) H, where the W+H is 3 semitones
Melodic Minor: Up: WHWWWWH Down: WHWWHWW
While interesting we will not practice this method of construction and instead focus on augmenting the major scale.
Construction Method 2
Using sets of intervals you can also construct minor scales directly:
Natural Minor: P1 M2 m3 P4 P5 m6 m7 P8
Harmonic Minor: P1 M2 m3 P4 m6 M7 P8
Melodic Minor: Up: P1 M2 m3 P4 P5 M6 M7 P8 Down: P8 m7 m6 P5 P4 m3 M2 P1
Again we will not practice this version.
Construction Method 3
This uses the circle of 5ths and 4ths just like we did for major scales. There is a catch however, since the natural minor scale is the only scale that shares the same intervals as the major scale it is the only one constructable from this method. You cannot make harmonic and melodic minor scales with this method.
I will not repeat the method here, instead if you want to know it go to the major scale lesson and see construction method 3. There is only 1 difference between the major and minor version. In the major scales C major is the starting point because it has no sharps or flats. For the natural minor version you start at A because A minor has no sharps and no flats. That is the only difference.
Practice
Using any method you like, construct D natural minor, A harmonic minor, B harmonic minor, G melodic minor, Bb harmonic minor, Ab natural minor, Eb harmonic minor, and C natural minor.
To support this series please consider donating via
paypalor joining the
patreon.