Major Scales

Goal

Be able to construct major scales. Understand the basic terms around scale degrees.

Scales

A scale is a collection of intervals. A scale can also be seen as a collection of notes if we are talking about a specific scale, such as C Major. Scales can be seen as a hierarchy that gives notes different “jobs”. We are going to focus on major scales in this lesson and how to construct them.

This is one of those topics that if you play an instrument it is obvious why scales are useful. They let you practice important patterns that you will see often. In music theory however scales are multi-layered beasts. The first layer of understanding is just being able to construct them.

Constructing Major Scales

Constructing Major Scales has 3 main ways that I am going to show you.

Construction by Steps
Construction by Intervals
Construction by Circle of 5ths or 4ths

Construction Method 1

Scales can be constructed from whole steps and half steps. To begin creating a scale, pick a starting note. Then from that starting note use the pattern WWHWWWH. Each W stands for a whole step and each H stands for a half step.

Universal Step 1

There is an important rule that you must always follow. You must always use each line and space only once for major scales. No line or space on the staff should represent 2 notes in the scale and no line or space should represent no notes! Each line and space gets 1 note for major scales!

Therefore the first step is always to fill in each line and space, this will let you create scales with the correct accidentals. Otherwise you risk writing an enharmonic equivalents that will sound the same but not be the right note!

Example

For example, let's pick the note D. D is the starting note. Therefore our first step is to write a note in every line and space from one D to the D an octave up.

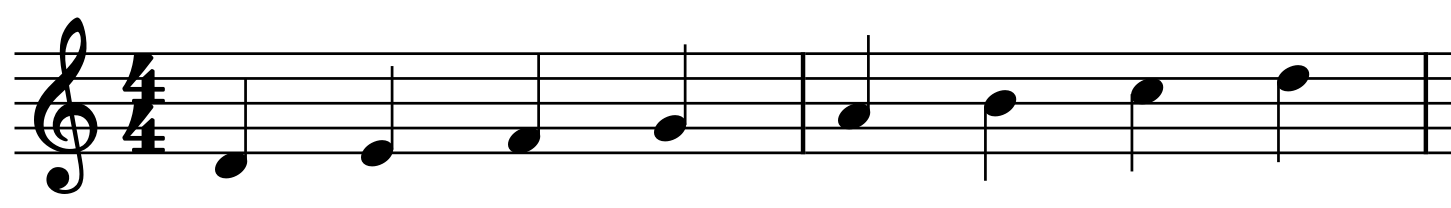
Now, and only now, do you apply the formula. Recall it is WWHWWWH. Starting at D, moving a whole step up is E. We see that E is already on the staff, so that note is approved.

Next we are at E and move the next whole step up. This gives us a black key. It can be F# or Gb. Since the next note must be an F (from universal step 1) we must pick the F# version.

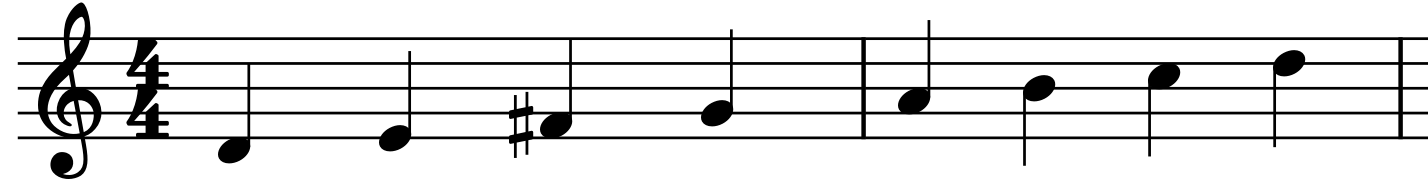
From F# we now must to the next part of the formula, a half step. This gives us the note G which we got from step one so this note is approved!

From G we have another whole step, giving us A, which we already have.

Next from A is another whole step giving B.

From B another whole step gives C# or Db. We already have a C so we pick C#.

Finally from C# we go one more half step to give D.

Therefore the final scale is: D E F# G A B C# D

Final Result

Note that every line and space rule makes us pick the same accidentals consistently! Major scales will always have only flats or only sharps. This is why notes like E# and B# exist or why sometimes a double flat or double sharp is required! It keeps an order to the system so that when you are working with a scale you know exactly what to expect!

Trickier Example

Let's do a scale with a lot of sharps to show how this guides us in picking the accidentals. Consider the scale F# major. Following the steps you should reach this result:

F# G# A# B C# D# E# F#

This scale contains an E#! This is because when you get to D# you will need another whole step which will land you a F natural. The problem is the note given to you from step one (the every line and space rule, or universal step one rule) is an E! So we must sharp the E to get the note we need.

Try it out!

Using this method I invite you to try and build the scales A major, Bb major, E major, and F major. You will notice that some scales have all flats and others have all sharps. This is a property of major scales that you can rely on.

Reveal Answer

Construction Method 2

Using half steps and whole steps is a bit of a tedious way to make scales. There exists better ways, so here is construction method 2.

A major scale is called a major scale because it contains mostly major intervals! (A more full discussion would require talking about major modes which are too advanced for us quite yet.) A major scale in particular has all major and perfect intervals. (But to be clear there are other major modes that have some minor intervals in them). So again we use the universal step 1, which is you pick a note and fill each line and space to the next octave up. (See construction method 1 if you skipped to here).

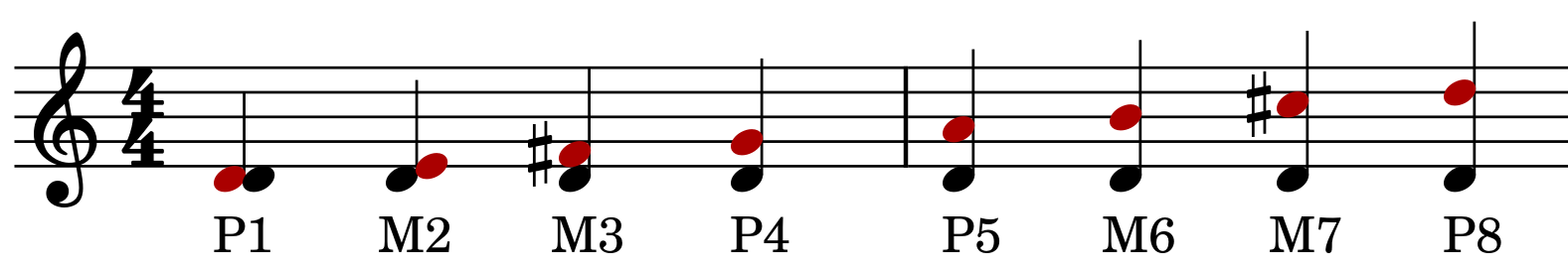
Now that we have the notes we must use we must check to make sure they are the right intervals. Note the major scale will always give you 7 notes since there are 8 notes in an octave. The fancy word for a 7 note scale is heptatonic. Hepta means 7, and tonic means they are constructed from the tonic note, which is the first note.

This means we will always have a 1st, 2nd, 3rd, 4th, 5th, 6th, and 7th in the scale. The major scale dictates that these must be perfect or major, so for a major scale we get the formula:

P1, M2, M3, P4, P5, M6, M7, P8

These intervals are all referenced to the tonic note, or starting note, also sometimes called the root note.

Example:

Consider D major again. Following the universal step 1 we must put a D down and write a note on every line and space to the next D. Since we are starting on D, D is said to be the tonic or root note. All intervals are constructed off of D. The first note must be a perfect first from D, which is D.

The second note must be a major 2 or M2 from D which is E.

The third note must be a major 3 or M3 from D which is F#. (Again here universal step one tells us what accidental we need.)

The fourth note must be a perfect 4 or P4 from D which is G.

Doing this for all the intervals yields the same scale as before. This construction method makes the intervals obvious and will give more understanding to the later concepts we will approach.

Try it out!

Using this method construct: Eb, E, Ab, A.

Reveal Answer

Construction Method 3

Video Explanation

This method relies on a pattern that occurs. There is a special pattern called the circle of 5ths and another one called the circle of 4ths.

The Circle of 5ths

It turns out that if you make a major scale on C you get no sharps and no flats, just natural notes. If you then go up a 5th from C you reach G. If you make a scale on G you get exactly 1 sharp in the scale! It turns out to be F#.

If you now go up a P5 from G you get to the note D. If you construct a major scale on D you get 2 sharps! F# and C#! Detecting a pattern?

It turns out that if you go up a 5th and make a major scale you will find that each time you gain 1 more sharp!

There comes a point where instead of talking in sharps we start using flats because there would be way too many sharps. Here is the complete circle:

-Source: Wikipedia

Going through this we get a special pattern known as the order of sharps. Each time we moved up a 5th we gained a new sharp and kept all the old sharps.

C - none

G- F#

D- F#C#

A- F#C#G#

E- F#C#G#D#

B- F#C#G#D#A#

F#- F#C#G#D#A#E#

C#- F#C#G#D#A#E#B#

At C# or F# we switch to using flats because double sharps would become required.

For this reason the order of sharps is: FCGDAEB. If a major scale needs 2 sharps they will be F and C. If it needs 3 the sharps will be F C G. If we can find how many sharps are required we can easily find which notes should be sharp!

An Important Discovery:

Look closely at the order of sharps. Notice anything? If you really look at it and compare it to the circle of 5ths you will find that it lines up with the circle of 5ths! C goes to G goes to D etc…

Using this realization now consider that C has no sharps, G has 1. Using the order of sharps we can tell right away that it is F!

Example

Let's say we want to know how many sharps are in A. Well, starting at C we have no sharps, then we move to G which has 1, then to D which has 2 so A must have 3! Using the order of sharps they must be F C and G!

In this way you can simply do step one and then use the order of sharps alone to create the required sharps!

But wait there's more! We can extend this system to work for flats as well!

The Circle of 4ths

The same thing happens for flats if you go in reverse! If you instead go backwards around the circle you will find that you are moving in fourths instead of fifths. C goes to F goes to Bb to Eb to Ab etc…

-Source: Wikipedia

And like before if you make a scale on each one you will find that C has no flats, F has 1, Bb has 2 etc…

C - none

F - Bb

Bb - Bb Eb

Eb - Bb Eb Ab

Ab - Bb Eb Ab Db

Db - Bb Eb Ab Db Gb

Gb - Bb Eb Ab Db Gb Cb

Cb - Bb Eb Ab Db Gb Cb Fb

Again as before at Gb you should consider switching to the sharp variant F#, definitely at Cb as B has far fewer accidentals as a sharp key then Cb does as a flat key!

The order of flats becomes clear:

BEADGCF

I remember it as the word “BEAD” + “Greatest Common Factor”.

Notice here something very interesting! Again the circle of 4ths is built right in just like the circle of 5th was built into the order of sharps but what's even more interesting is the order of flats backwards is the order of sharps!

This means if you know one, you know them both! So if you can count and know the order of flats or the order of sharps then you can make any major scale. (We will see this same system can be used on the natural minor scales as well!)

I always start with the order of flats. Here are the rules you need to keep in mind:

If you move in the same direction of flats then you must use flats.
If you move in the same direction as the order of sharps then you must use sharps.
Looping around adds the word “sharp” or “flat” to the scale name depending on the direction you were going.
If the word sharp or flat is in that name then you must move in that direction.
If the word sharp or flat is not used then you should go directly to that letter without looping around an edge.

Example:

Consider the scale Bb.

First we write the order of flats: BEADGCF.

Next we must find how many sharps or flats Bb has. Since a flat is already in the name we must move in the same direction as the order of flats. Starting at C we go to F and then loop around to B.

Looping around gives the word “Flat” to the name of the scale. (If you were to loop more than once you would have double flat and triple flat etc…) So now we have reached Bb. We moved 2 spots to get to Bb from C (since C has no flats or sharps) therefore Bb must have 2 flats! (Since we moved in the direction of flats). The first 2 flats in the order of flats are Bb and Eb, therefore we can now make the scale.

First follow universal step 1:

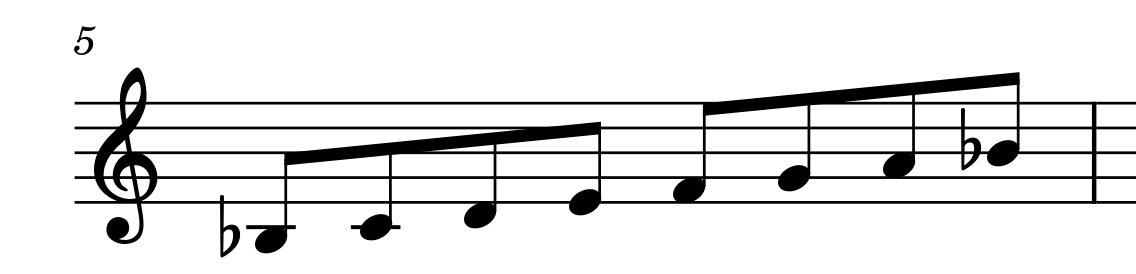
Now apply the flats:

It's that easy.

Example 2

Consider the scale F# major.

Write the order of flats BEADGCF. We again will count starting from C. Note that the word sharp is in the name so we must move in the order of sharps direction. Doing this we go through all the letters and then loop around to F confirming that we are now at F sharp major. We have 6 sharps. This means that the first 6 letters in the order of sharps must be used.

First follow universal step 1:

Now apply the sharps:

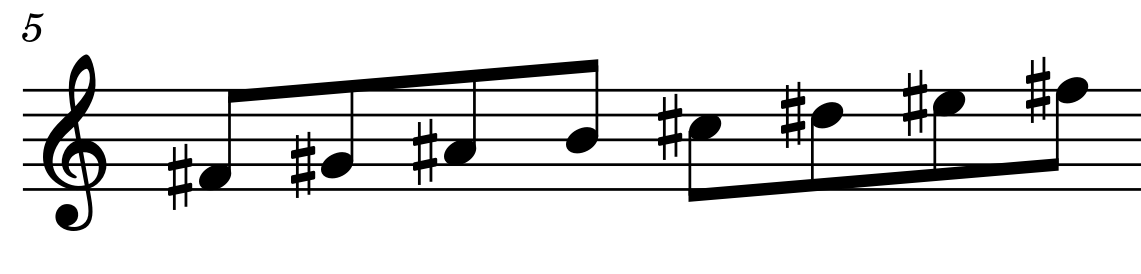
Example 3

Consider the scale E major.

First we write the order of flats BEADGCF. Here no flat or sharp is in the name so we start at C (again because it has no sharps or flats) and go right to E without looping because the scale name didn't have a flat or sharp in it so we go straight to it without going off an edge. Doing this we go from C to G to D to A to E. We have moved 4 spots so it will need 4 sharps. We know we must use sharps because we have moved in the sharp direction. Using the order of sharps we see the the first 4 sharps F#, C#, G#, and D#.

First follow universal step 1:

Now apply the sharps:

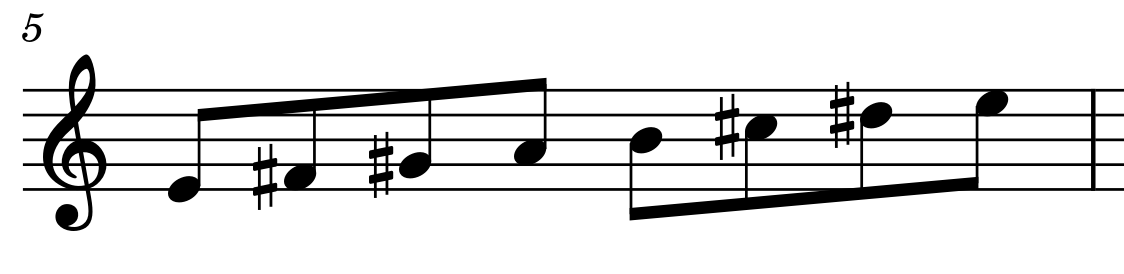
Example 4 Looping More than Once

What if we have a scale that requires us to loop more than once? First, such scales are almost never needed, you should consider an equivalent simpler scale in most cases. For now let's consider the scale D## major.

We write the order of flats BEADGCF. Sharp is in the name so we move in the sharp direction. Starting at C we go: C->G->D (At this point we are only at D major)->A->E->B->LOOP POINT (scales now have the word sharp in their name only once) F->C->G->D (We are only at D# major)->A->E->B->LOOP POINT (scales now have the word sharp in their name twice) -> F->C->G->D (We finally reach D## major).

We see now that we moved 16 times! So 16 sharps are needed! To do this we note that we looped 2 times, therefore all the notes are at least sharped twice. This takes care of 14 of the sharps. Next we have 2 left, starting at the order of sharps that means F and C must be triple sharps! The scale would look crazy.

First follow universal step 1:

Now apply the sharps:

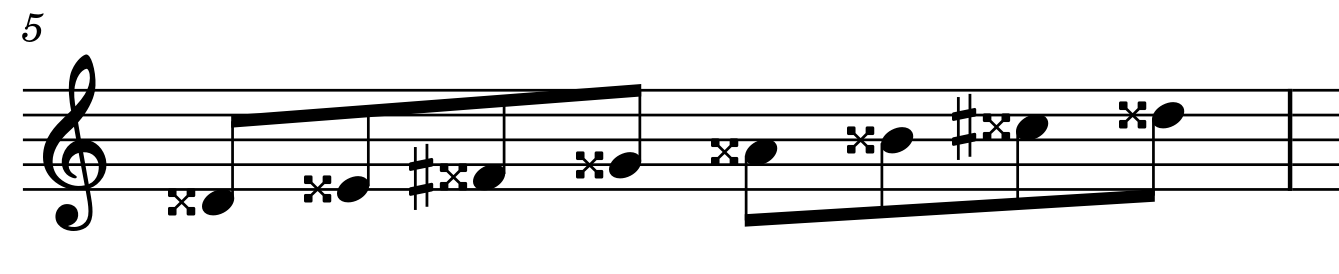
Look at this more closely and realize this scale looks like D major! Only 2 notes are really genuinely sharped. For example if we made D####, we would find that all the notes are quadruple sharped except for F and C which will have quintuple sharps! It turns out this is true for all these crazy major scales! It would sound like E major but it is written so it looks like D major. For this reason we don’t ever come across these but if you understand what I am pointing out then it means you have a strong grasp on the order of sharps/flats and how it relates to the circle of 5ths/4ths.

Try it out

Using this method construct the following major scales: Ab, Eb, Gb, Fb, Cb, D, E, A, B, F#, C#.

Reveal Answer

To support this series please consider donating via

paypal

or joining the

patreon.